12/12/2016
Scientists from the Institute of Physics and Technology of the Russian Academy of Sciences and MIPT have let two electrons loose in a system of quantum dots to create a quantum computer memory cell of a higher dimension than a qubit (a quantum bit). In their study published in Scientific Reports, the researchers demonstrate for the first time how quantum walks of several electrons can help to implement quantum computation.
“By studying the system with two electrons, we solved the problems faced in the general case of two identical interacting particles. This paves the way toward compact high-level quantum structures,” comments Leonid Fedichkin, Expert at the Russian Academy of Sciences, Vice-Director for Science at NIX (a Russian computer company), and Associate Professor at MIPT’s Department of Theoretical Physics.

While a classical 3-bit register encodes three variables, a quantum register of 3 qubits encodes eight.
In a matter of hours, a quantum computer would be able to hack through the most popular cryptosystem used even in your web browser. As far as more benevolent applications are concerned, a quantum computer would be capable of molecular modeling that takes into account all interactions between the particles involved. This in turn would enable the development of highly efficient solar cells and new drugs. To have practical applications, a quantum computer needs to incorporate hundreds or even thousands of qubits. And that is where it gets tricky.

The qubit is the basic element of a quantum computer. It has two basis states, viz., |0? and |1?. The distinction between the classical bit and its quantum counterpart lies in more than just fancy brackets (these are the standard quantum mechanical notation for a state). The essential property of a qubit is its ability to be in a superposition of the two basis states: A|0?+B|1?. A classical bit, on the other hand, can only have one of the two values (0 or 1). The term "qudit" can be used to refer to higher-level quantum systems with more than two states.
CREDIT: MIPT's Press Office
The qubit is the basic element of a quantum computer. It has two basis states, viz., |0⟩ and |1⟩. The distinction between the classical bit and its quantum counterpart lies in more than just fancy brackets (these are the standard quantum mechanical notation for a state). The essential property of a qubit is its ability to be in a superposition of the two basis states: A|0⟩+B|1⟩. A classical bit, on the other hand, can only have one of the two values (0 or 1). The term “qudit” can be used to refer to higher-level quantum systems with more than two states.
As it turns out, the unstable nature of the connection between qubits remains the major obstacle preventing us from using quantum walks of particles for quantum computation. Unlike their classical analogs, quantum structures are extremely sensitive to external noise. To prevent a system of several qubits from losing the information stored in it, liquid nitrogen (or helium) needs to be used for cooling. Plenty of schemes have been proposed for the experimental realization of a separate qubit. In an earlier study, a research team led by Prof. Fedichkin demonstrated that a qubit could be physically implemented as a particle “taking a quantum walk” between two extremely small semiconductors known as quantum dots, which are connected by a “quantum tunnel.” From the perspective of an electron, the quantum dots represent potential wells. Thus, the position of the electron can be used to encode the two basis states of the qubit—|0⟩ and |1⟩—depending on whether the particle is in one well or the other. Rather than sit in one of the two wells, the electron is smeared out between the two different states, taking up a definite position only when its coordinates are measured. In other words, it is in a superposition of two states.
If an entangled state is created between several qubits, their individual states can no longer be described separately from one another, and any valid description must refer to the state of the whole system. This means that a system of three qubits has a total of 8 basis states and is in a superposition of them: A|000⟩+B|001⟩+C|010⟩+D|100⟩+E|011⟩+F|101⟩+G|110⟩+H|111⟩. By influencing the system, one inevitably affects all of the 8 coefficients, whereas influencing a system of regular bits only affects their individual states. By implication, n bits can store n variables, while n qubits can store 2ⁿ variables. Qudits offer an even greater advantage, since n four-level qudits (aka ququarts) can encode 4ⁿ, or 2ⁿ×2ⁿ variables. To put this into perspective, 10 ququarts store approximately 100,000 times more information than 10 bits. With greater values of n, the zeros in this number start to pile up very quickly.
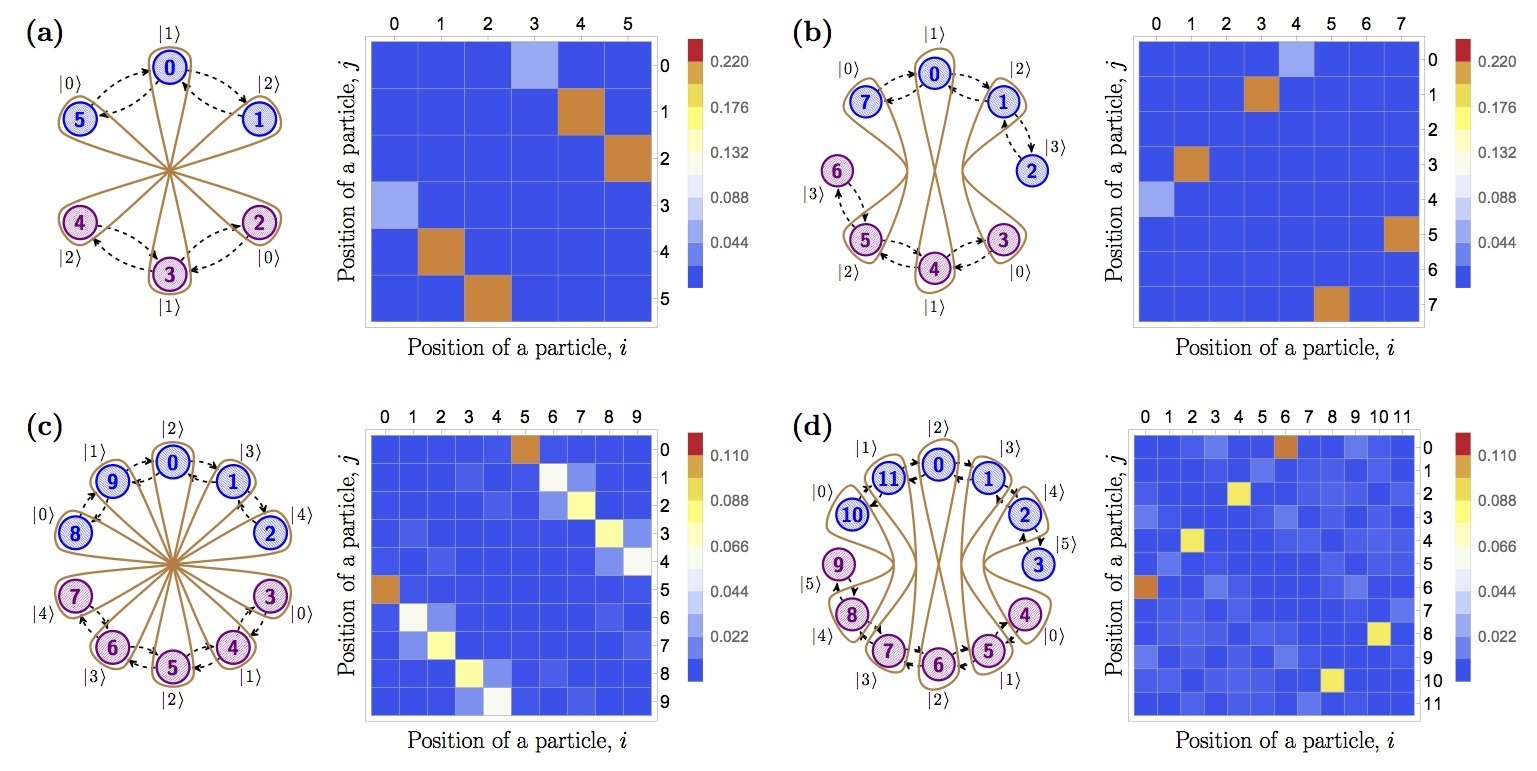
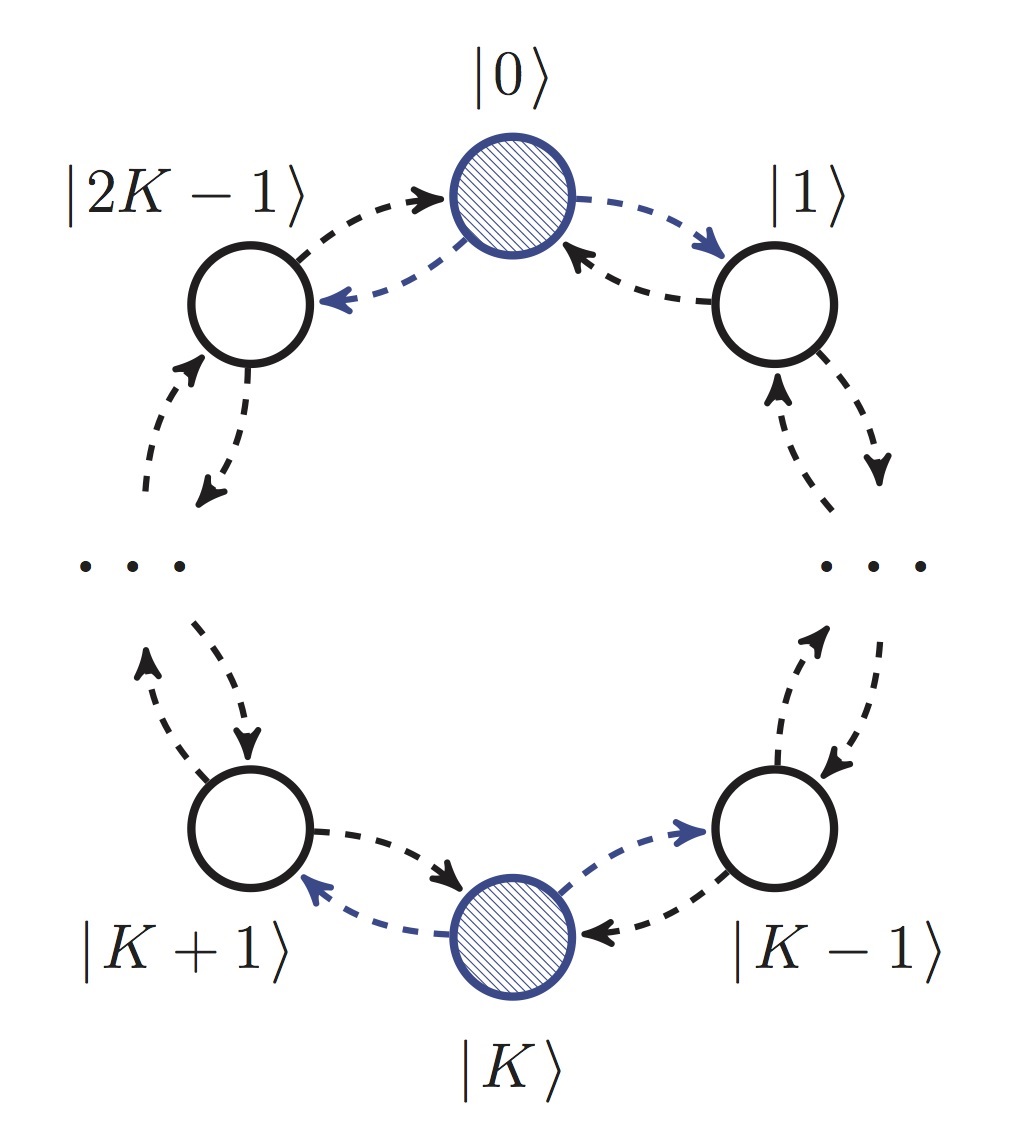
In this study, Alexey Melnikov and Leonid Fedichkin obtain a system of two qudits implemented as two entangled electrons quantum-walking around the so-called cycle graph. To make one, the scientists had to “connect the dots” forming a circle (once again, these are quantum dots, and they are connected by the effect called quantum tunneling). The entanglement of the two electrons is caused by the mutual electrostatic repulsion experienced by like charges. It is possible to create a system of even more qudits in the same volume of semiconductor material. To do this, it is necessary to connect quantum dots in a pattern of winding paths and have more wandering electrons. The quantum walks approach to quantum computation is convenient because it is based on a natural process. Nevertheless, the presence of two identical electrons in the same structure was a source of additional difficulties that had remained unsolved.
The blue and purple dots in the diagrams are the states of the two connected qudits (qutrits and ququarts are shown in (a) and (b) respectively). Each cell in the square diagrams on the right side of each figure (a–d) represents the position of one electron (i = 0, 1, 2, … along the horizontal axis) versus the position of the other electron (j = 0, 1, 2, … along the vertical axis). The cells color-code the probability of finding the two electrons in the corresponding dots with numbers i and j when a measurement of the system is made. Warmer colors denote higher probabilities.
The phenomenon of particle entanglement plays a pivotal role in quantum information processing. However, in experiments with identical particles, it is necessary to distinguish so-called false entanglement, which can arise between electrons that are not interacting, from genuine entanglement. To do this, the scientists performed mathematical calculations for both cases, viz., with and without entanglement. They observed the changing distribution of probabilities for the cases with 6, 8, 10, and 12 dots, i.e., for a system of two qudits with three, four, five, and six levels each. The scientists demonstrated that their proposed system is characterized by a relatively high degree of stability.
It has been a long time since people first set their hearts on building a universal quantum computer, but so far we have been unable to connect a sufficient number of qubits. The work of the Russian researchers brings us one step closer to a future where quantum computations are commonplace. And although there are algorithms that quantum computers could never accelerate, others would still benefit enormously from devices able to exploit the potential of large numbers of qubits (or qudits). These alone would be enough to save us a couple of thousand years.