February 26, 2016

Today’s atomic clocks are ridiculously accurate. The best of them tell time so well that if they had been running since the Big Bang, by now they would not have gained or lost more than a second.

But scientists are always looking to improve their time-keepers, for benefits to GPS, communications, internet applications, and all the measurements that rely on time (including the meter). One frustration that keeps atomic clocks from becoming more accurate is fundamental noise that’s tied to the quantum nature of atoms.  

Researchers from NIST’s Physical Measurement Laboratory (PML) and JILA, the joint research and training institute run by NIST and the University of Colorado Boulder, are conducting proof-of-principle work to reduce this quantum noise that appears in all quantum sensors, with potential use someday for increasing the precision of not just atomic clocks but for many other applications including inertial sensors, gravimetry, and electromagnetic field sensing.

The world’s most precise atomic clocks use vacuum-pumped chambers of atoms such as cesium, strontium, or ytterbium. First, the atoms are energized with a probe light that puts some of them into a higher energy state, while the rest remain in the ground state. That starts the clock hand ticking.* However, the quantum nature of the atoms creates a fundamental noisiness in their time-keeping capabilities.

“In an atomic clock, the quantum noise in your telling of the time is analogous to having a clock hand that is fundamentally fuzzy,” says PML/JILA’s James Thompson, who is leading the work. “If I average many measurements of my clock, I would find out that it’s pointing at, say, 2 o’clock. But on any single try, I would find that sometimes it’s pointing a little past 2 o’clock and sometimes a little before 2 o’clock. This makes it difficult to tell the time precisely without making many measurements.”

One way to get around this fundamental quantum fuzziness, Thompson explains, is to entangle the atoms. Entanglement describes a relationship where each atom in the clock, in a sense, knows what all the other atoms are doing.

“And if you create the right type of entanglement – we call this type of entanglement spin squeezing – it turns out the atoms can conspire with each other, so that the noisiness or the fuzziness associated with one atom is partially canceled by the fuzziness of another atom,” Thompson says. “This is a little like making the width of the clock hand narrower at the expense of more fuzziness in the length of the clock hand – a direction that is not important for telling the time.”

So far Thompson’s team has demonstrated that they can squeeze the “quantum fuzziness” out of the value of interest by about a factor of 60 in noise variance.

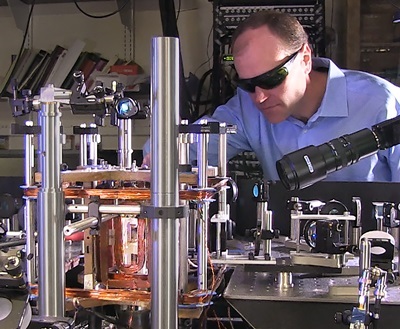
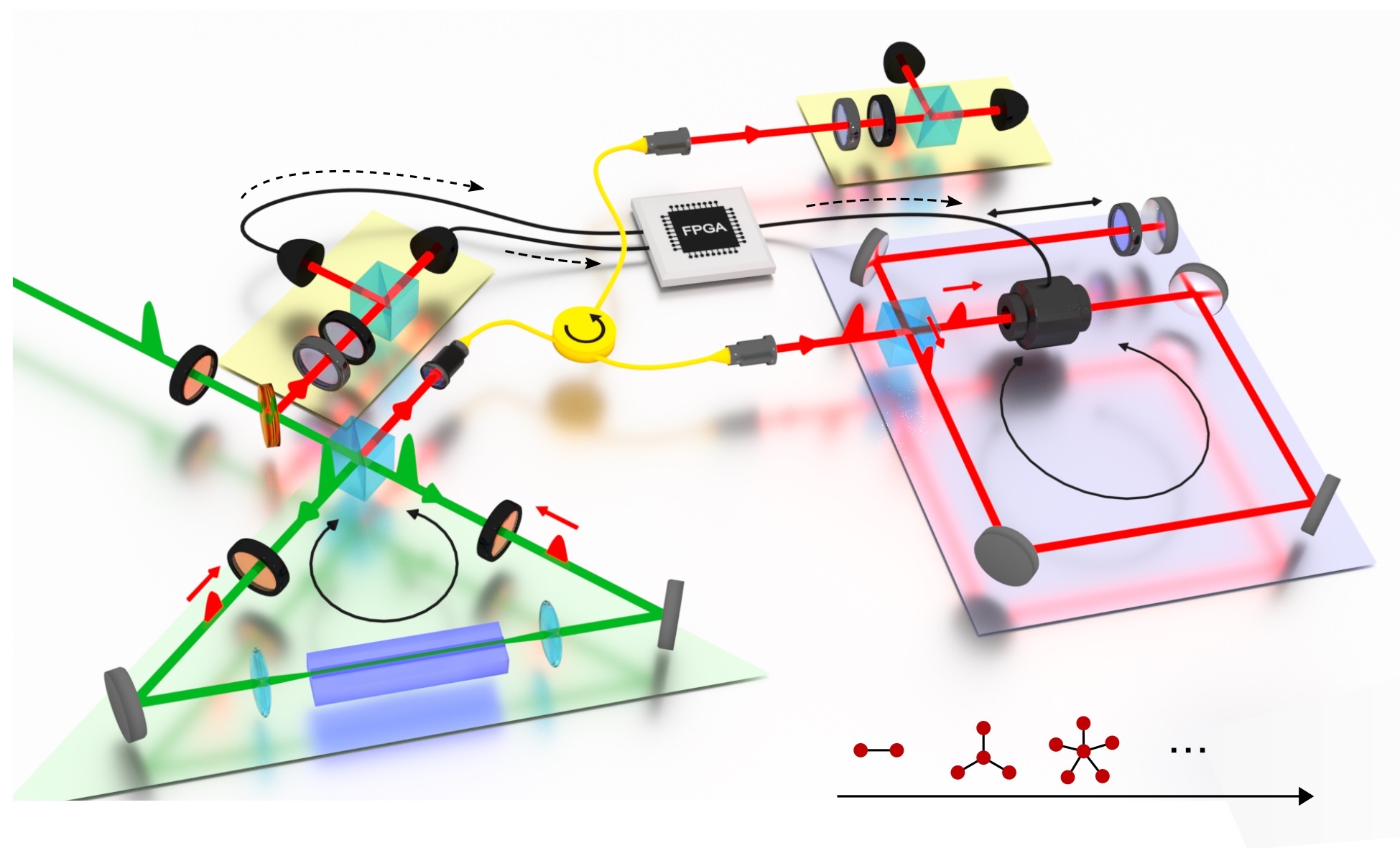
In their experiment, approximately 1 million rubidium atoms are kept in vacuum and cooled to just 10 microkelvin, or ten millionths of a kelvin above absolute zero. The researchers levitate the atoms in an optical lattice, a grid of intersecting beams that creates a kind of egg carton for holding the atoms. This setup keeps the atoms extremely still, so that they don’t move during the measurements. It also isolates the atoms from the rest of the world, “so that they can live in a world where quantum mechanics is important,” Thompson says.

The rubidium atoms are suspended in an optical cavity, between two almost perfectly reflective mirrors. The spacing of the mirrors determines the cavity’s resonance frequency, similar to how the size of a bell determines the note at which it rings. Then, just as with an atomic clock, the atoms are partially energized to an excited state.

The trick to making a measurement with this setup is that the atoms in an excited state change the frequency of the cavity’s “note.”

“Each atom in the excited state acts like a piece of glass in between the two mirrors. The index of refraction inside the glass is different from vacuum, and therefore it would change the wavelength of light at is passes through that glass,” Thompson explains. “And that would be equivalent to effectively changing the mirror spacing. That would shift the resonant frequency.”

The tuning of the cavity’s ringing can be determined by shining a red laser light at one of the cavity mirrors and measuring the properties, specifically the phase, of the reflected light. By asking only this question – how does the resonant frequency of the cavity change? – the researchers were able to tease out the answer to real question they wanted to ask, which was how many atoms are likely to be in one state versus the other.**

Quantum noise causes the number of atoms in the excited state to fluctuate, but the measurement reveals what value it has taken on any given trial. From the tuning of the cavity, the experimenters can tell how many atoms are in the excited state, but not which atoms are in the excited state. This keeps each atom in both the excited and ground states, a requirement for the clock to be able to tick after the measurement.

“What’s amazing is that simply the act of measuring this information forces the atoms into an entangled state,” says Kevin Cox, a graduate student and member of Thompson’s lab. “Each atom remains in both an excited energy state and an unexcited energy state at the same time, yet the total number of excited atoms is precisely known.”

The work doesn’t necessarily translate directly to an atomic clock measurement yet. However, many potential applications are possible with further development. The current accuracy of the strontium clock is about 2 parts in a billion billion. With this technique, Thompson says, that precision could go up by a factor of 10.

“For the scale that we’re talking about, that’s a huge win,” Thompson says.

Their quantum noise reduction technique could also potentially be used to increase the sensitivity of rotation sensors, accelerometers, magnetometers, gravity sensors, and atom interferometers, which can be used for a range of applications including GPS-free navigation and measurements of gravitational waves and the gravitational constant.

“It’s exciting because in some sense people have been working with the idea of entanglement for quantum computation, information, and communication,” Thompson says. “The idea of entanglement is kind of a toy to be played with thus far.” What his team has shown, he continues, is that this is a real tool that could potentially reduce noise in measurements with quantum sensors. “That’s what we wanted to see: Is this a real technique or not? And it looks like it very well might be.”

—Jennifer Lauren Lee

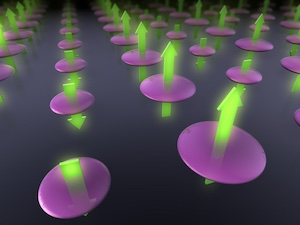
A Visualization of Squeezing: A quantum system, such as a collection of rubidium atoms, can be represented visually with a Bloch sphere. Points on the surface of the sphere correspond to states of the system, and the position of each point is given by two angles, which represent related properties that cannot be measured to high accuracy simultaneously. However, due to the Heisenberg uncertainty principle, there is some fundamental fuzziness in the exact position of each point. Using entanglement, physicists can squeeze the uncertainty out of the position of a point in one dimension, at the expense of certainty in a perpendicular dimension.
Measuring the orientation of the Bloch vector (position of the arrow in this animation) is at the heart of all quantum sensors with broad applications for time keeping, definition of SI units, gravimetry, and inertial and electromagnetic field sensing.

*An atomic clock doesn’t have hands like a clock on a mantelpiece, but physicists often use the clock-hand visualization in technical descriptions. The international standard (SI, Système International) definition of a second is a certain number of transitions (about nine trillion) between two states of a cesium atom.

**What they gave up, due to Heisenberg uncertainty, was knowledge about a collective phase of the atoms. But they didn’t need that for these measurements.

The Heisenberg uncertainty principle says that you can’t know both the position and momentum of a particle at the same time with high certainty. (If you measure one of those values, the uncertainty for the other goes through the roof.)