28 November 2013
It is fascinating how quantum mechanical behaviour of particles at smallest scales can give rise to strange properties that can be observed in the classical world. One example is the Fractional Quantum Hall Effect (FQH) that was discovered about 30 years ago in semiconductor devices. It is one of the most striking phenomena in condensed matter physics and has been thoroughly investigated. Nowadays experimental physicists are able to model effects occurring in condensed matter with ultracold atoms in optical lattices. This has sparked the interest in the question under which conditions the FQH could be observed in such systems. Now Anne Nielsen and co-workers from the Theory Division of Professor Ignacio Cirac at the Max-Planck-Institute of Quantum Optics and at the Universidad Autónoma de Madrid have developed a new lattice model which gives rise to FQH-like behaviour (Nature Communications, 28 November 2013).
The classical Hall-effect describes the behaviour of electrons or, generally spoken, charge carriers in an electrical conducting probe under the influence of a magnetic field that is directed perpendicular to the electric current. Due to the Lorentz-force a so-called Hall-voltage builds up, which increases linearly with the magnetic field.
In 1980 the German physicist Klaus von Klitzing investigated the electronic structure of so-called MOSFETs. At extremely low temperatures and extremely high magnetic fields he made the discovery that the Hall-resistivity would rise in small steps where the inverse of each plateau was an integer multiple of a combination of constants of nature. A few years later probes of gallium-arsenide, investigated under similar conditions, showed additional plateaus that would correspond to fractional multiples. These discoveries gave a completely new insight into the quantum mechanical processes that take place in flat semiconductor devices, and both were awarded with the Nobel prize in Physics: in 1985 the Nobel prize was given to Klaus von Klitzing, in 1998 to Robert Laughlin, Horst Störner and Daniel Tsui.
The FQH effect is a fascinating phenomenon and explained by theoreticians as being caused by one or more electrons forming composite states with the magnetic flux quanta. However, detailed experimental investigations of FQH are difficult to do in solids, and the states are very fragile. A cleaner implementation could be obtained by realizing the phenomenon in optical lattices in which ultracold atoms play the role of the electrons. This, and the hope to find simpler and more robust models displaying the FQH, is why theoreticians around the world seek to understand which mechanisms could lead to the observation of the FQH in lattices.
To this end the MPQ-team sets the focus on the topological properties that FQH states have. The topology of an object represents certain features of its geometrical structure: For example, a tee cup with a single hole in the handle and a bagel are topologically equivalent, because one can be transformed into the other without cutting it or punching holes in it. A bagel and a soccer ball, on the other hand, are not. In solid state systems the electrons experience the electric forces of many ions that are arranged in a periodic structure. Usually their energy levels make up straight and continuous energy ‘bands’ with a trivial topology. Instead, in systems that exhibit the fractional quantum Hall effect, the topology provides the material with exotic properties, like that the current can only be transmitted at the edge and is very robust against perturbations.
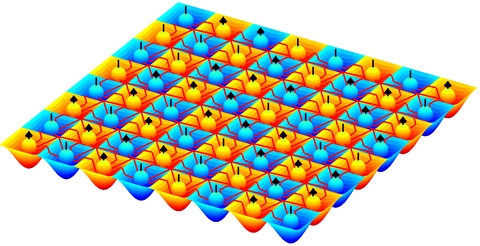
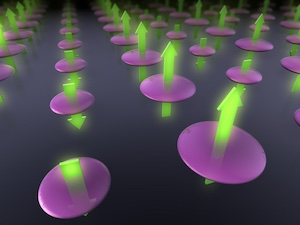
“We have developed a new lattice model where a FQH state should be observed,” Anne Nielsen says, first author of the publication. “It is defined on a two-dimensional lattice in which each site is occupied with a particle. Each particle can be either in a ‘spin up’ or a ‘spin down’ state. In addition, we imply specific, local, short range interactions between the particles.” (See figure 1.) Numerical investigations of the properties of this system showed that its topological behaviour is in accordance with the one expected for a FQH state. The system does, for example, possess long range correlations that lead to the presence of two different ground states of the system when considering periodic boundary conditions.
To obtain their model the researchers used some specific mathematical tools. These tools are by themselves interesting because they may be more widely applicable and thereby open up doors to construct further interesting models.
“The mechanism that leads to FQH in our model seems to be different from those in previous models”, Anne Nielsen points out. “And, furthermore, we have demonstrated how this model can be implemented with ultracold atoms in optical lattices. Realizing FQH states in optical lattices would give unique possibilities for detailed experimental investigations of the states under particularly well-controlled conditions and would, in addition, be a hallmark for quantum simulations.” Olivia Meyer-Streng
Original publication
Anne E. B. Nielsen, Germán Sierra, and J. Ignacio Cirac
Local models of fractional quantum Hall states in lattices and physical implementation
Nature Communications, 28 November 2013, DOI: 10.1038/ncomms3864